03. Find Square Root

Ex 1: For Positive Numbers

# Python Program to calculate the square root

# Note: change this value for a different result
num = 8

# uncomment to take the input from the user
#num = float(input('Enter a number: '))
num_sqrt = num ** 0.5
print('The square root of %0.3f is %0.3f'%(num ,num_sqrt))

Output: The square root of 8.000 is 2.828

In this program, we store the number in num and find the square root using the ** exponent operator. This program works for all positive real numbers. But for negative or complex numbers, It will not work.

Ex 2: For Negative Numbers or Complex Numbers

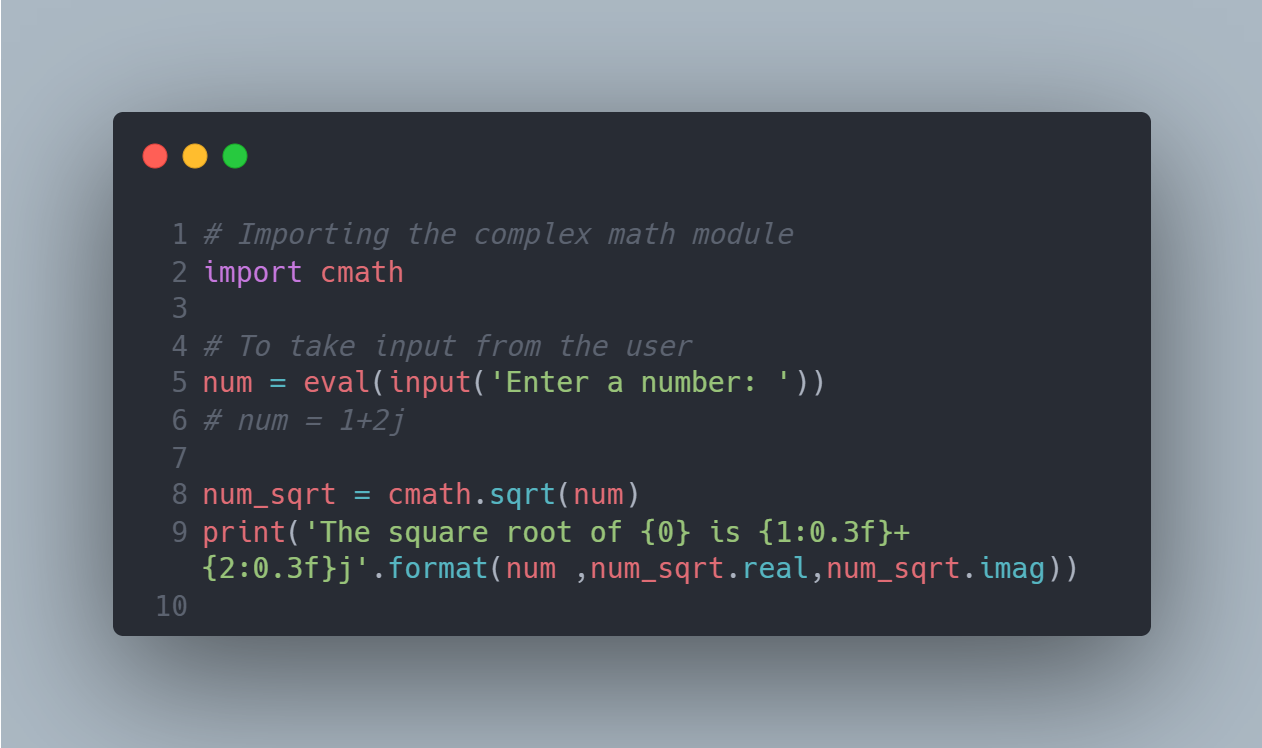
# Python Program to calculate the square root

import cmath

# Take input from the user
num = eval(input('Enter a number: '))
# num = 1 + 2j

num_sqrt = cmath.sqrt(num)
print('The square root of {0} is {1:0.3f}i + {2:0.3f}j'.format(num, num_sqrt.real, num_sqrt.imag))

Output:

Enter a number: -16

The square root of -16 is 0.000+4.000j

Enter a number: 16

The square root of 16 is 4.000+0.000j

The square root of (1+2j) is 1.272+0.786j

Enter a number: 3+4j

The square root of (3+4j) is 2.000+1.000j

In this program, we use the sqrt() function in the cmath (complex math) module.

Note: If we want to take complex number as input directly, like 3+4j, we have to use the eval() function instead of float().

The eval() method can be used to convert complex numbers as input to the complex objects in Python. To learn more, visit Python eval() function.

Ex 3: Using F String

import cmath

num = 1 + 2j

num_sqrt = cmath.sqrt(num)
print(f'The square root of {num_sqrt} is {num_sqrt.real: 0.3f} + {num_sqrt.imag: 0.3f}j')

Output: The square root of (1.272019649514069+0.7861513777574233j) is 0.786+0.786j